
It was in 1994 that MIT professor of applied mathematics Peter Shor developed a groundbreaking quantum computing algorithm capable of factoring numbers using quantum computer technology.
Shor's algorithms here, provided a glimpse of the potential power of quantum computers. Using qubits instead of bits, quantum computers in theory, should be a lot faster than traditional classic computers. But to prove that, researchers have spent decades of experimenting and researching.
And even with such long time, researchers could never prove quantum would always be faster in this application, or whether classical systems could overtake quantum if given a sufficiently robust algorithm of its own.
Researchers were scrambling and wondering, until IBM found something.
The IBM researchers Sergey Bravyi, David Gosset and Robert König said in their Quantum Advantage With Shallow Circuits paper:
"Our work gives an unconditional proof of a computational quantum advantage and simultaneously pinpoints its origin: It is a consequence of quantum nonlocality. The proposed quantum algorithm is a suitable candidate for near-future experimental realizations, as it requires only constant-depth quantum circuits with nearest-neighbor gates on a two-dimensional grid of qubits (quantum bits).”
In the paper published by the team, shows that quantum algorithms can solve problems in a fixed number of steps, regardless of how many inputs are added. This is a contrast to classical computers, which adds more steps to solve when the system is given more inputs.
"The main point of this paper is not that somehow we discover some incredibly important quantum algorithm, or some practical, interesting problem," explained Bravyi. "We ask if we can separate a constant depth [between] quantum and classical algorithms. As we increase the problem size, the runtime of the quantum algorithm remains constant, but the total number of operations grows."
In short, quantum computers need only a fixed number of steps to solve a problem, even as the number of inputs increases. This makes quantum computation faster than the classical counterpart, because the more complex the problem is, the more efficient the quantum computed solution would be.
Related: The First Time Researchers Found Something That Only Quantum Computers Can Solve
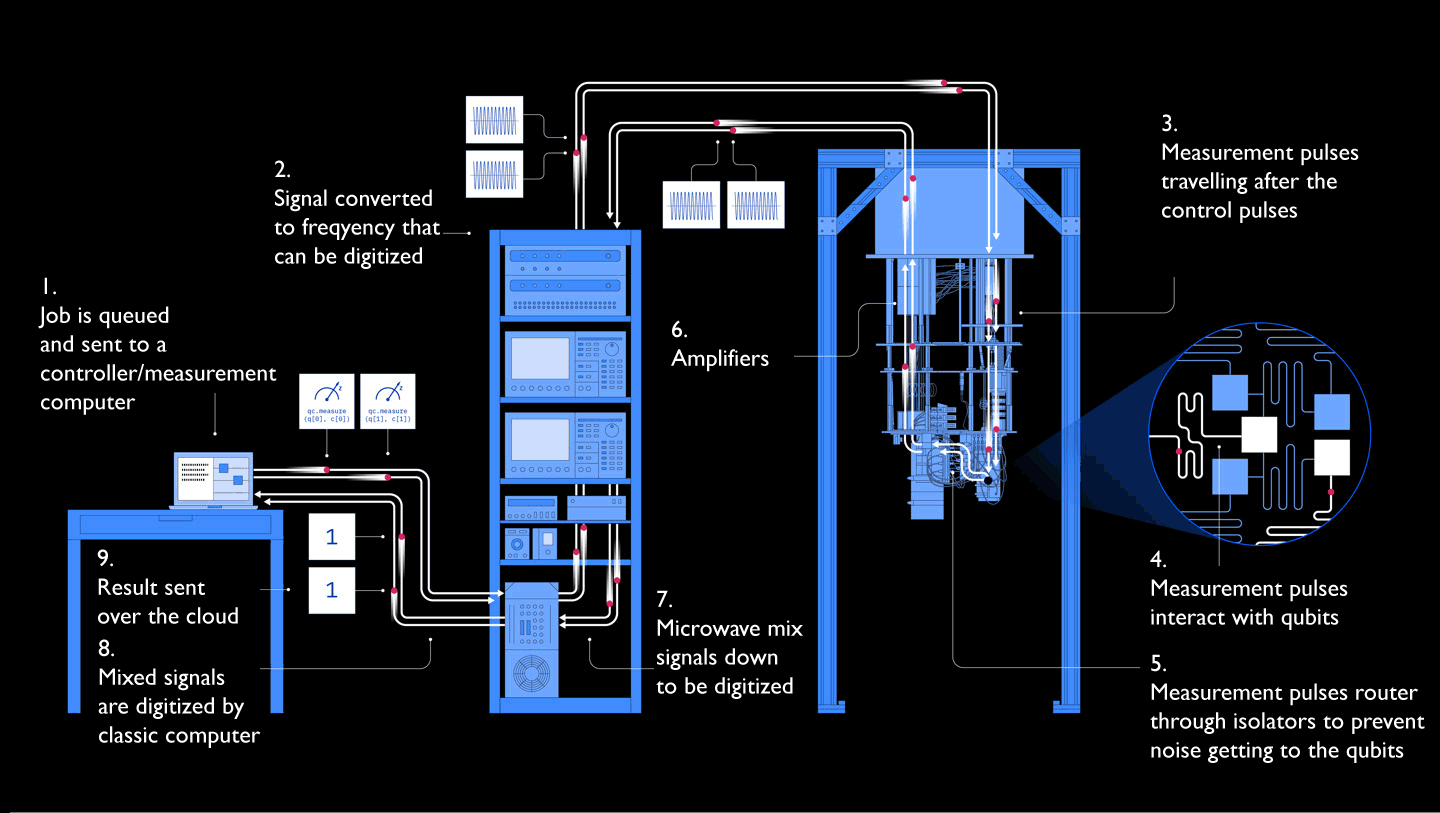
With this result, algorithms can be developed for quantum computers, which can also be made to improve hybrid classical-quantum systems as well.
"We can start discussing things to a much greater depth than we had to, or were able to, before. We can start to really kind of separate out for people, what goes in to all the decisions about creating quantum computers, and creating the software stack on them, and algorithms."
IBM in proving that quantum computers can indeed be more efficient than classical ones, mark a milestone towards quantum supremacy.
IBM and other tech companies, like Google, Microsoft, Intel and others are already in a race against each other to make this happen. The underlying hardware for quantum computers should be further developed to become more powerful. There is also the problem with qubits which aren't yet perfect, as they still have small error rates, and they can only exist for certain length before becoming chaotic.
The proof by the researchers doesn't, and and of itself, solve any existing computation issues. Instead, "it gives us insight into what makes a quantum computers more powerful," the researcher continued. "And hopefully in the future it will lead to more practical, useful algorithms."
it's until that time we solve quantum computing issues that quantum technologies can really prove itself capable of solving problems faster than any supercomputer on Earth...